Maths question
Discussion
The missus is (re)sitting her Maths GCSE and did a class last night. They are on powers/indices. She was confused by a question and my answer was different to the teacher's. The teacher said their answer was correct.
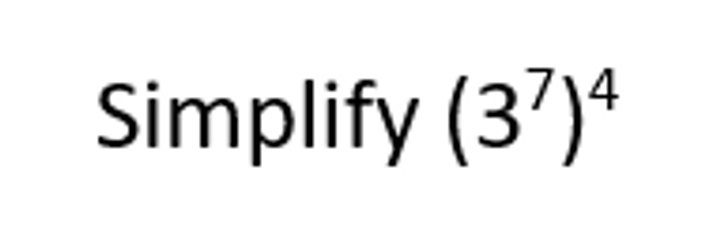
I said: Just multiply 7 and 4 so the answer is 3^28 (3^7 x 3^7 x 3^7 x 3^7)
The maths teacher said: You have to raise 3^4 as well i.e. 3x3x3x3, as well as multiplying the indices, so their answer was 81^28
I'm definitely correct aren't I?
I said: Just multiply 7 and 4 so the answer is 3^28 (3^7 x 3^7 x 3^7 x 3^7)
The maths teacher said: You have to raise 3^4 as well i.e. 3x3x3x3, as well as multiplying the indices, so their answer was 81^28
I'm definitely correct aren't I?
Wolfram Alpha is a handy tool for checking things like this
https://www.wolframalpha.com/input?i2d=true&i=...
https://www.wolframalpha.com/input?i2d=true&i=...
(yes, you are correct)
https://www.wolframalpha.com/input?i2d=true&i=...
https://www.wolframalpha.com/input?i2d=true&i=...
(yes, you are correct)
Panamax said:
I am absolutely not a mathematician.
My recollection is "deal with what's inside the bracket first" so it looks to me as though the teacher's right.
Now expand the brackets correctly and count up the 3sMy recollection is "deal with what's inside the bracket first" so it looks to me as though the teacher's right.
(3x3x3x3x3x3x3) x (3x3x3x3x3x3x3) x (3x3x3x3x3x3x3) x (3x3x3x3x3x3x3)
How many do you get?
Like I said, I'm not a mathematician. However, I was taught many years ago to solve the bracket first. On this basis I felt the "4" should be applied to the answer to the bracket - not just apply a bunch more "3"s.
Anyway, I'm happy to guided by experts! Just don't let me near the controls....
Anyway, I'm happy to guided by experts! Just don't let me near the controls....
Panamax said:
Like I said, I'm not a mathematician. However, I was taught many years ago to solve the bracket first. On this basis I felt the "4" should be applied to the answer to the bracket - not just apply a bunch more "3"s.
Anyway, I'm happy to guided by experts! Just don't let me near the controls....
Dealing with the bit in brackets first and that result to the power 4 is the same answer as 3 to the power 28.Anyway, I'm happy to guided by experts! Just don't let me near the controls....
Panamax said:
Like I said, I'm not a mathematician. However, I was taught many years ago to solve the bracket first. On this basis I felt the "4" should be applied to the answer to the bracket - not just apply a bunch more "3"s.
Anyway, I'm happy to guided by experts! Just don't let me near the controls....
The brackets are superfluous really, they're just there for clarity so you don't think it's 3^74Anyway, I'm happy to guided by experts! Just don't let me near the controls....
The power laws are very simple, and if you forget them you can work them out again from scratch.
Also, as the example is fully numeric, you can check it with a calculator and see that the teacher is wrong.
Agreed and understand why the question is about simplifying a problem. But there’s no way that question would have been on my GCSE paper.
Maybe if it was it would have been a good thing. GCSEs were piss easy pass in my day.
I can do laplace transforms and dfts in my head but irrelevant other than to say can do maths and looked at that question thinking why is it a question rather than what’s the answer.
Maybe if it was it would have been a good thing. GCSEs were piss easy pass in my day.
I can do laplace transforms and dfts in my head but irrelevant other than to say can do maths and looked at that question thinking why is it a question rather than what’s the answer.
Stupid teacher.
You could of course simplify a bit more:
3^28 = 9^14 = 81^7 = 22,876,792,454,961
It becomes more interesting if you leave the brackets out, in that case you raise 3 to the power of (4 to the power of 7):
3^16384 = a number with 7818 digits
(if you really want to know: approximately 1.4277012078932958292545728220963e+7817)
You could of course simplify a bit more:
3^28 = 9^14 = 81^7 = 22,876,792,454,961
It becomes more interesting if you leave the brackets out, in that case you raise 3 to the power of (4 to the power of 7):
3^16384 = a number with 7818 digits
(if you really want to know: approximately 1.4277012078932958292545728220963e+7817)
Edited by Jim1064 on Thursday 17th October 18:49
Edited by Jim1064 on Thursday 17th October 20:17
Edited by Jim1064 on Thursday 17th October 20:17
Gassing Station | Science! | Top of Page | What's New | My Stuff




 king imbecile. I’d be asking for a different teacher.
king imbecile. I’d be asking for a different teacher.