Maths/Probability Question
Discussion
OK, here's the scenario.
You're taking part in a game. There are 10 boxes and 10 contestants. 1 box contains the prize, the other 9 are empty. Contestants pick their box and open it, 1 at a time, in full view of others. There was a draw to see who decides the box opening order, and you won. You can choose if you open a box first, last, or anywhere in between.
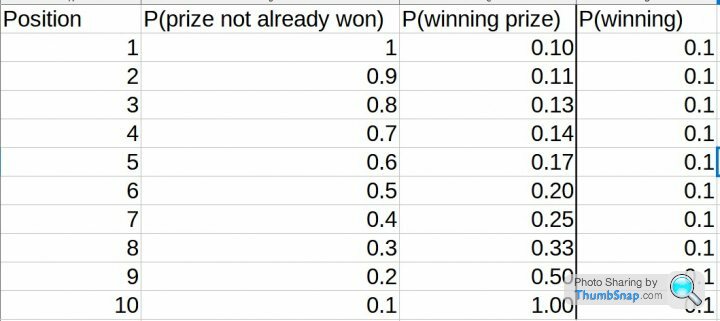
If you go first, you are guaranteed a chance to compete for the prize, but only have a 1 in 10 chance of winning. If you go last, you are guaranteed to win if you get to take your turn, but there's a 9 in 10 chance one of the others will have won it already, and the game is then over. Go 2nd, and your chances of winning are increased to 1 in 9, but there's a 1 in 10 chance the person who went first picked the winning box so you never get your turn. Go 6th, and there's now a 1 in 5 chance of winning (20%), but a 5 in 10 (50%) chance that the game will be over before it gets to you, as one of the first 5 has already won.
So, what's the best position. 1st, last or somewhere in the middle. Gut feeling tells me it makes no difference. The longer you leave it, the greater your chance of picking the winning box, but the greater your chance of seeing someone else win before you even get a go.
Thoughts?
You're taking part in a game. There are 10 boxes and 10 contestants. 1 box contains the prize, the other 9 are empty. Contestants pick their box and open it, 1 at a time, in full view of others. There was a draw to see who decides the box opening order, and you won. You can choose if you open a box first, last, or anywhere in between.
If you go first, you are guaranteed a chance to compete for the prize, but only have a 1 in 10 chance of winning. If you go last, you are guaranteed to win if you get to take your turn, but there's a 9 in 10 chance one of the others will have won it already, and the game is then over. Go 2nd, and your chances of winning are increased to 1 in 9, but there's a 1 in 10 chance the person who went first picked the winning box so you never get your turn. Go 6th, and there's now a 1 in 5 chance of winning (20%), but a 5 in 10 (50%) chance that the game will be over before it gets to you, as one of the first 5 has already won.
So, what's the best position. 1st, last or somewhere in the middle. Gut feeling tells me it makes no difference. The longer you leave it, the greater your chance of picking the winning box, but the greater your chance of seeing someone else win before you even get a go.
Thoughts?
MDMA . said:
My view is once you have picked your box, you have a one in ten chance of winning. It doesn’t matter if you go first, middle or last. It’s still one in ten as the winning box has never changed hands. The odds never change.
You don't pick your box until it's your go. If you go first it's 1 in 10. But if you go last, there's only 1 box left, say No3 where the prize is, so you have a 100% chance of winning. But of course, going last, there's a 90% chance someone else will have picked no3 and won, so the game ends and you never even get to have a go. Edited by TwigtheWonderkid on Friday 28th April 17:23
I think it's a little more complex.
You get to choose where you are in the order of contestants.
When it's each contestant's turn, they then pick a box and open it (assuming the winning box hasn't already been opened).
So let's say the other contestants are A to I.
If you don't go first, contestant A picks one of the ten boxes and opens it.
If it's empty, either you jump in and open a box at random, or contestant B does.
And so on.
You get to choose where you are in the order of contestants.
When it's each contestant's turn, they then pick a box and open it (assuming the winning box hasn't already been opened).
So let's say the other contestants are A to I.
If you don't go first, contestant A picks one of the ten boxes and opens it.
If it's empty, either you jump in and open a box at random, or contestant B does.
And so on.
MesoForm said:
It's been a long week so this could well be completely wrong, but isn't in 10% chance of winning no matter what position you're in?
Yes, that's my thinking, but as with the Monty Hall problem, I thought perhaps the answer wasn't as obvious as I might think on first glance. 
If it is 10% regardless, then going first is the best thing to do. It doesn't improve your chances, but it guarantees you the excitement of opening a box.
Edited by TwigtheWonderkid on Friday 28th April 17:26
TwigtheWonderkid said:
MesoForm said:
Yes, that's my thinking, but as with the Monty Hall problem, I thought perhaps the answer wasn't as obvious as I might think on forst glance. Yours remains 1 in 10.
TwigtheWonderkid said:
MesoForm said:
Yes, that's my thinking, but as with the Monty Hall problem, I thought perhaps the answer wasn't as obvious as I might think on forst glance. SpeckledJim said:
The chance you have the right box is 1 in 10. It doesn’t matter when anyone else opens their 1 in 10 box. Now. Yesterday. Tomorrow.
Yours remains 1 in 10.
Your chance of winning may be 10%, but as you don't pick your box until it's your go, the chances of having the right box is only 10% if going first. If you go 6th, your chance of picking the right box is 1 in 5, as there are only 5 left, so that's 20%. Of course, there's a 50% chance you won't get to pick a box at all going 6th, as the prize has been won by 1 of the first 5. Yours remains 1 in 10.
TwigtheWonderkid said:
Your chance of winning may be 10%, but as you don't pick your box until it's your go, the chances of having the right box is only 10% if going first. If you go 6th, your chance of picking the right box is 1 in 5, as there are only 5 left, so that's 20%. Of course, there's a 50% chance you won't get to pick a box at all going 6th, as the prize has been won by 1 of the first 5.
In which case you have a 50% of 20%chance, ie 10%cheesejunkie said:
The big difference with the Monty Hall one is that you don't get to re-chose as I'm sure you're aware.
Well you kind of do. You might start out deciding to go in the middle, 6th, and pick box C (if boxes are A-J). But box C gets picked by 1 of the first 5, so you have to choose another when it's your go. If you get to have a go at all. Super Sonic said:
TwigtheWonderkid said:
Your chance of winning may be 10%, but as you don't pick your box until it's your go, the chances of having the right box is only 10% if going first. If you go 6th, your chance of picking the right box is 1 in 5, as there are only 5 left, so that's 20%. Of course, there's a 50% chance you won't get to pick a box at all going 6th, as the prize has been won by 1 of the first 5.
In which case you have a 50% of 20%chance, ie 10%I'm a bit of a dullard when it comes to maffs so please be gentle.
I don't understand how it's a 10% chance whichever box you choose to open.
Say you choose to open the 7th box (so 6 have been opened and 4 remain) - the previous 6 opening events don't count anymore as they've already happened. You go for opening the 7th and at that point you've a 1 in 4 // 25% chance of success?
I.e. your chances of success are only 10% at the point where you choose (or not) to open the first box?
I am sure I am missing something obvious.
I don't understand how it's a 10% chance whichever box you choose to open.
Say you choose to open the 7th box (so 6 have been opened and 4 remain) - the previous 6 opening events don't count anymore as they've already happened. You go for opening the 7th and at that point you've a 1 in 4 // 25% chance of success?
I.e. your chances of success are only 10% at the point where you choose (or not) to open the first box?
I am sure I am missing something obvious.
tomw2000 said:
I'm a bit of a dullard when it comes to maffs so please be gentle.
I don't understand how it's a 10% chance whichever box you choose to open.
Say you choose to open the 7th box (so 6 have been opened and 4 remain) - the previous 6 opening events don't count anymore as they've already happened. You go for opening the 7th and at that point you've a 1 in 4 // 25% chance of success?
I.e. your chances of success are only 10% at the point where you choose (or not) to open the first box?
I am sure I am missing something obvious.
They do count.I don't understand how it's a 10% chance whichever box you choose to open.
Say you choose to open the 7th box (so 6 have been opened and 4 remain) - the previous 6 opening events don't count anymore as they've already happened. You go for opening the 7th and at that point you've a 1 in 4 // 25% chance of success?
I.e. your chances of success are only 10% at the point where you choose (or not) to open the first box?
I am sure I am missing something obvious.
If you decide to take the 7th box there’s a 60% chance that by the time we get to you the winner has already been found.
Whichever way you dice it, it’s ultimately 10%.
It doesn’t matter when the boxes get opened. 1 wins and 9 lose.
Gassing Station | Science! | Top of Page | What's New | My Stuff




